Séminaire de Mécanique d'Orsay
Le Jeudi 12 mars 2020 à 14h00 - Salle des séminaires FAST-LPTMS (Bât. 530, salle C.120, 1er)
A growth model driven by curvature reproduces geometric features of arboreal termite nests
Giulio FACCHINI
Life Sciences Department, University of Roehampton, London, UK
We present a simple three-dimensional model to describe the autonomous expansion of a substrate which grows driven by the local mean curvature of its surface. The model aims to reproduce the nest construction process in arboreal Nasutitermes termites , whose cooperation may be similarly mediated by the shape of the structure they are walking on, for example focusing the building activity of termites where local mean curvature is high. We adopt a phase-field model where the nest is described by one continuous scalar field and its growth can be described with a single non-linear equation with one adjustable parameter d. When d is large enough the equation is linearly unstable and fairly reproduces a growth process where the initial walls expand, branch and merge, while progressively invading all the available space, which is consistent with the intricate structures of real nests (cf. Fig. 1). Interestingly, the linear problem associated to our growth equation is analogous to the buckling of a thin elastic plate under in-plane compression which is known to produce rich patterns through non linear and secondary instabilities . We validate our model by collecting nests of two species of arboreal Nasutitermes from the field and imaging their structure with a micro-CT scanner. We find a strong resemblance between real and simulated nests, characterised by the emergence of a characteristic length-scale and by the abundance of saddle-shaped surfaces with zero-mean curvature which validates the choice of the driving mechanism of our growth model.
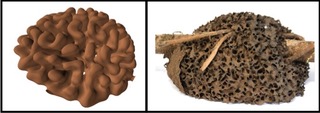
Figure 1: Numerical simulation of our growth model (left) and fragment of an arboreal nest of Nasutitermes ephratae collected from the field (right)
Accès Salle des séminaires FAST-LPTMS (Bât. 530, salle C.120, 1er)
