Séminaire de Mécanique d'Orsay
Le Jeudi 3 juillet à 14h00 - Salle de conférences du FAST
Two-frequency excitation of single-mode Faraday waves
William Batson
Technion-Israel Institute of Technology, Haifa, Israel
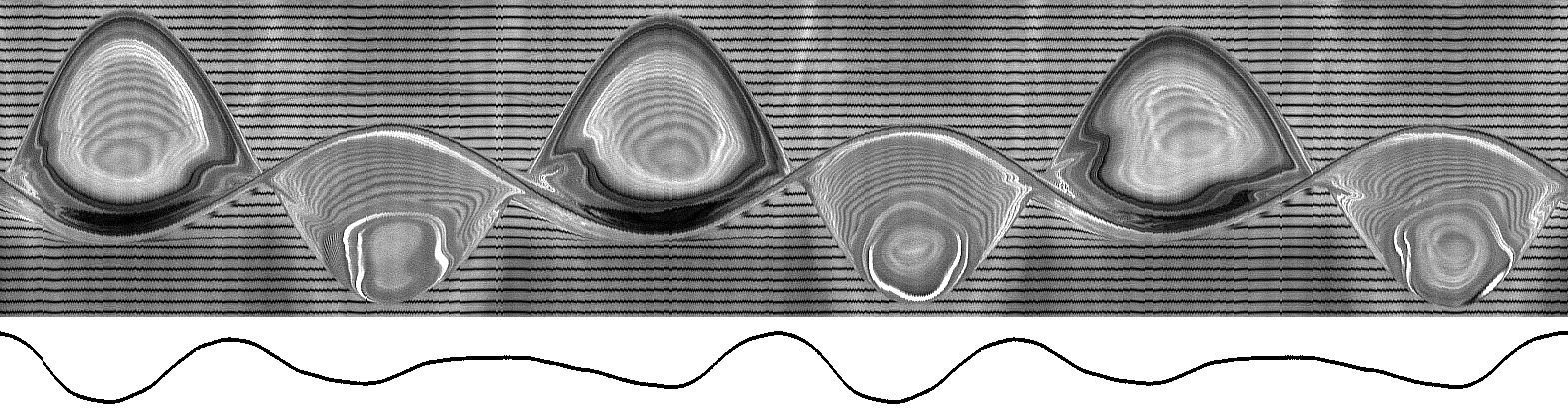
To date, two-frequency excitation of the Faraday instability has been considered only in the high-frequency regime, typically in the interest of unlocking patterns that are unavailable to single-frequency excitation. In this work, we consider such excitations in the low-frequency regime, where the mode selection is discretized and cell modes can be individually excited. The course of study follows that of Batson et al. [1], who designed and compared a cylindrical, single-mode experiment with the linear theory of Kumar & Tuckerman [2], to which a stress-free sidewall boundary condition was applied to effect mode discretization. This boundary condition was successfully approximated by judicious choice of the experimental fluids, and, as a result, remarkable agreement was observed between the predicted thresholds and the experimental onsets. A central question to this work therefore is whether or not the same agreement is seen for two-frequency excitation. The investigation utilizes the two-frequency linear theory of Besson et al. [3], to which the stress-free boundary condition is again applied. With a substantially increased parameter space owing to the introduction of three degrees of freedom–amplitude, frequency, and phase of the second component-focus is restricted to the case where the two frequencies are of the same order. The model is then used as a guide to explore basic phenomena in the experiment of Batson et al: Most notably, the model predicts that a second frequency can produce either significant or insignificant adjustment to the single component threshold amplitude. Whether or not this occurs depends on the frequency ratio, and three different ratios are studied to experimentally verify this prediction. In the nonlinear regime, bifurcation and time series data of the saturated wave response (see linked figure) are used to differentiate the two-frequency and single-frequency cases. Finally, a novel multi-mode excitation, whereby both frequency components excite a cell mode, is highlighted.
References:
[1] W. Batson, F. Zoueshtiagh and R. Narayanan, The Faraday threshold in small cylinders and the sidewall nonideality, J. Fluid Mech. 729, 496–523 (2013).
[2] K. Kumar, L. S. Tuckerman, Parametric instability of the interface between two fluids, J. Fluid Mech. 279, 49–67 (1994).
[3] T. Besson, W. S. Edwards and L. S. Tuckerman, Two-frequency parametric excitation of surface waves, Phys. Rev. E 54, 507–514 (1996).